Srinivasa Ramanujan was the strangest man in all of
mathematics, probably in the entire history of science. He has been compared to a bursting supernova,
illuminating the darkest, most profound corners of mathematics, before being
tragically struck down by tuberculosis at the age of 33, like Riemann before him.
- Michio Kaku
G
H Hardy (Ramanujan’s mentor) contrived an informal scale of mathematical
ability on which he assigned himself a 25 and Littlewood a 30. To David Hilbert, the most eminent
mathematician of the day, he assigned an 80.
To Ramanujan he gave 100.
- Quoted by Robert
Kanigel, Ramanujan’s biographer
Preamble
This year is the 125th
birth anniversary of the great Indian mathematician Srinivasa Ramanujan.
The nation is celebrating it as the Year of Mathematics. Also, his birth date, December 22, has been
declared as the National Mathematics Day in apparent similarity to February 28
that is being observed since 1986 as the National Science Day in tribute to the
Nobel Prize winning contribution of physicist C V Raman [See my earlier blog
post: 45) Commemorating a historical achievement –
Raman his effect and the National Science Day (Feb 12)]
Ramanujan’s advent on the mathematical stage 125 years ago
was not only like the explosion of a supernova as Michio Kaku put it but also nearly
as rare in a field that boasts the likes of Archimedes, Newton, Euler and
Gauss. Considering that the last great
supernova in our (Milkyway) galaxy happened as far back as 1604, mathematics is
perhaps fortunate with a much higher frequency of such exploding talent as exemplified
by Ramanujan and his likes. His
appearance would have been dazzling enough anywhere in the developed world, but
the fact that it happened in a little known place in an obscure and subjugated country,
not particularly visible in the intellectual firmament, was infinitely more so.
Though supernovae flare up and fade away rapidly, they leave
behind them remnants of the event that are of lasting significance and keep
astronomers busy for decades and even centuries as is the case with the famous
Crab Nebula, the remnant of a supernova that erupted in 1054 AD. Similar is the case with infrequently erupting
mathematical geniuses who keep lesser mathematicians busy studying, analyzing,
and building upon their contributions for decades to come. The story of Srinivasa Ramanujan of India is
a classic example of this.
A star is born
The birth of Ramanujan on 22 Dec 1887 to a very poor and highly
orthodox Hindu Brahmin family in a sleepy town in South India was as
insignificant an event as anything can be.
Like the overwhelming majority of stars in the night sky, this one was
invisible and would have remained so but for the circumstances that made it
explode into a bright supernova soon and spend itself in the process. His father, a sales assistant in a textile
shop in Kumbakonam, was a virtual non-entity throughout his life while his
mother, a domineering woman, had a very significant influence on his upbringing
and future. She nursed him through an
attack of the dreaded small pox that devastated the region when he was just two. He completed his primary education with
flying colors when ten, standing first in the district, and joined the Town
High School in Kumbakonam where he exhibited exceptional brilliance in
Mathematics. He was lent a book on
advanced trigonometry by S L Loney which he not only mastered quickly but also
made many sophisticated discoveries on his own.
He continued to excel in academic studies and received a much needed
scholarship to pursue further education at the government college in
Kumbakonam.
Soon came his sensational tryst with destiny in the form of a
well-known book on Mathematics – A
Synopsis of Elementary Results in Pure and Applied Mathematics, by the
English mathematician G S Carr. It
produced such a magical and obsessive influence on the young man that he found
himself immersed in it most of his wakeful hours, to the exclusion of other
academic pursuits. He not only mastered
its contents in a matter of months, but went on to go far beyond their scope
through discoveries of his own that Carr could never have imagined. This was perhaps the first spark of the
supernova that would soon draw compelling attention in the mathematical
firmament.
Agony amidst ecstasy
Carr’s Synopsis had
ignited such a burst of fiercely single-minded and unassisted intellectual
activity in him that he lost interest in everything else. He put in only a physical presence in his classes
and was always lost in mathematical thought without paying any attention to the
lessons. This started reflecting in his
academic performance in other subjects to such an extent that he actually
failed in most of them. An inevitable
consequence was the loss of his scholarship and a life of extreme poverty and
dependence on friends and relatives for livelihood. He would earn a little by giving tuitions in
mathematics to higher class students, but not many were attracted to this
because his world of mathematics was very different from theirs.
Unable to bear with the difficulties of life confronting him,
Ramanujan sought an escape from it by just running away from home to far off
Vishakhapatnam apparently in search of a change in his fortunes, but was soon
located and brought back home. He then
shifted to Madras and joined Pachaiyappa’s College where he again excelled in
mathematics and performed so poorly in other subjects that he failed once again
and had to give up formal studies. But
this drove him deeper into mathematics and into a world of his own where his
creative genius flourished even as he found himself on the verge of starvation
many a time.
Ramanujan’s mother decided that a solution to his misfortunes
lay in his marriage, to a nine-year old girl, consistent with the practice of
child marriages widely prevalent in Hindu society. His circumstances grew if anything even worse
and he went about looking for any sort of job in Madras to keep his body and
soul together. He would carry his
mathematical notebooks with him and introduce himself as someone with an
original attainment in the subject which he needed to pursue with a modest job
to provide essential livelihood. He was
such a simple, honest and likeable person that people went out of the way to
help him in various ways and was soon successful in his modest quest.
Light at the end of the
tunnel
Ramanujan was soon able to enlist the sympathies and support
of several well placed people most of whom were also mathematicians, though
involved in more lucrative professions.
Initially he got introduced to deputy collector Mr V Ramaswamy Iyer who
had recently founded the Indian Mathematical Society. The latter was quickly
struck by the extraordinary mathematical findings contained in Ramanujan’s
notebooks and he didn’t want to offer the young man a measly job in his office;
so he sent him looking elsewhere for better openings with letters of
introduction focusing on his mathematical prowess. With tremendous difficulty Ramanujan eventually
met Mr R Ramachandra Rao the district collector of Nellore and the secretary of
the Indian Mathematical Society. Ramachandra
Rao initially found it very difficult to believe that whatever he saw in the
notebooks was Ramanujan’s original work, but soon found overwhelming
circumstantial evidence to support the claim.
He was so impressed that he dug into his own personal resources to
provide some regular financial support for the young prodigy until he got a
suitable job.
Ramanujan was soon to end up as a ‘class III, grade IV
accounts clerk’ at a salary of rupees 30 per month in the Madras Port Trust
office and was happy with it considering that he had no college degree and the
job afforded sufficient spare time for his mathematical pursuits. More significantly, his immediate boss was Mr
S Narayana Iyer who was the treasurer of the Indian Mathematical Association
and an admirer. Also, the head of the
Port Trust itself was Sir Francis Spring who had also been tremendously
impressed with his mathematical researches.
Such contacts enabled him to get several of his research findings
published in the Journal of the Indian Mathematical Society, enhancing his reputation
as a mathematical genius of exceptional merit.
Earlier, one of the professional mathematicians who had encouraged him
greatly and appreciated his work was P V Seshu Iyer, a Professor at Pachaiyappa’s
College where Ramanujan had actually failed.
In search of
recognition
It soon dawned on friends and well-wishers of Ramanujan that
his raw talent could not grow to its full potential in Indian conditions and
needed the vastly superior intellectual environment that existed in Europe,
preferably England for understandable reasons.
Samples of his work were presented to a few eminent British
mathematicians for assessment and professional support, but met with little or
no response. Undaunted, Ramanujan was
advised to contact Professor G H Hardy at Cambridge, one of the most renowned
contemporary mathematicians. He did so
in January 1913, enclosing nine pages of his results as a ‘sample’. His covering letter said in part; “…I have not trodden through the… university
course, but am striking out a new path for myself. I have made a special investigation of
divergent series in general and the results I get are termed by the local
mathematicians as ‘startling’… Being
poor, if you are convinced that there is anything of value I would like to have
my theorems published…I have not given the actual investigations nor the
expressions I get but I have indicated the lines on which I proceed…” He was trying to invoke both pity and admiration
at the same time, but in effect was only seeking recognition of the
international mathematical community.
Ramanujan’s appeal to Hardy and the eventual outcome of it
was in many ways similar to Satyendranath Bose’s appeal to Albert Einstein much
later, seeking the latter’s help in getting his own path breaking work in
Quantum Physics published [See my earlier blog post: 30) Bose and Einstein – A Historic
Collaboration (Jul 11)]
Hardy’s Response
This time Ramanujan’s effort met with the kind of response he
was hoping for. Though stunned and
incredulous, even annoyed, with what appeared to him superficially as some
outrageous claims of a lowly unschooled clerk from the backwaters of the
British empire, Hardy had the patience and good sense to go through Ramanujan’s
notes in detail and come to the conclusion that they must be either an
elaborate hoax or the work of an incredibly gifted genius. He sought a second opinion from his great
friend and younger professional colleague, J E Littlewood, who also felt the
same way. With a mixture of perplexity
and amazement, Hardy concluded that Ramanujan’s claims ‘must be true because,
if they were not, no one could have the imagination to invent them’.
Replying to Ramnujan’s appeal, Hardy wrote very encouragingly
and, at the same time pointed out a few major flaws and deficiencies in his
findings that required further examination.
In particular, he complained that most of the findings, stated in the
form of end results without the intermediate steps involved in arriving at
them, required to be proved with the kind of rigour that was the hallmark of
mathematics as a discipline. He wanted
Ramanujan to send such proofs to buttress his claims. On his part, Ramanujan had not paid much
attention to such finer points, having very often arrived at the final results
through a gigantic leap of his intuition and imagination. Many a time he had indeed worked out some of
the more important intermediate steps, but only on a slate, and had not
transferred them into the written form for he couldn’t even afford the paper
required! In most cases they had been
spun out only in his mind, leaving the reader to figure out the details! As it emerged later, few could indeed do this
and it was left to other geniuses like Hardy and Littlewood to fill in the
gaps!
Invitation to Cambridge
The recognition he secured from Hardy and his associates at
Cambridge also meant that Ramanujan had gone up several notches in the eyes of
officialdom in Madras who were now willing to vie with each other in conferring
special privileges on him. The
University of Madras bestowed a special and generous scholarship on him with
full freedom to pursue his mathematical researches. With this, he was liberated from both his
gnawing poverty and the dependence on others.
Unknown to Ramanujan, Hardy had initiated steps to get him
invited to Cambridge to work with him and others at the famous Trinity
College. This was a great deal more
than Ramanujan had hoped from Hardy and initially refused to consider the idea
because of long held religious edicts that forbade orthodox Brahmins to ‘cross
the seas’ to go to foreign lands. Hardy
was disappointed to hear this and assigned the delicate task of persuading
Ramanujan to change his mind to his Cambridge colleague E H Neville who was
bound for Madras to give a series of mathematical lectures there. Before Neville could take up the issue with
him Ramanujan had received ‘divine intervention’ through the medium of his
mother lifting the objection to crossing the seas. Neville was pleasantly surprised to discover
that there was no need for any human intervention. The coast was now clear for Ramanujan to go
to Cambridge and show the world what he was worth.
Life and Work at
Cambridge
Ramanujan’s departure from Madras to England on 17 March 1914
was an event of considerable excitement and most of his friends and supporters
were on hand to see the prodigy off. The
ship’s captain promised to give him special treatment as long as he didn’t talk
mathematics with him!
Neville himself was on hand in England to receive him four
weeks later and see him settled in Cambridge after a brief acclimatization in
London.
Despite his humble origin and background, Ramanujan’s fame had
preceded his arrival in Cambridge and he was looked upon by most people in this
historic town as someone special. In
India, Neville had assured him much to his great relief that he would be
enrolled in the Trinity College as a research fellow and would not be required
to pass any examinations. He had little
difficulty in adjusting himself to Cambridge’s academic atmosphere and in
establishing a cordial working relationship with his great benefactor Hardy,
Littlewood and others. However, the same
could not be said of his personal life.
As a strict vegetarian with distinctly south Indian food habits and a teetotaler,
he faced considerable difficulties. Soon
he found it necessary to turn into a cook and prepare his own food in a
kitchenette attached to his living room.
P C Mahalanobis who later founded the Indian Statistical Institute in Calcutta
was of great help with some of his personal problems.
Hardy and Littlewood both got to examine all of Ramanujan’s notebooks
in detail and what they discovered was even more amazing than what they had
been led to believe from their previous correspondence with him. While any professional touch was
conspicuously absent in his writings, their authenticity and originality was never
in question. Here was someone without
any formal education beyond high school coming up with some of the most
astounding discoveries in the entire history of mathematics, a realization that
could not have come to anyone but people of their own caliber. Some of Ramanujan’s discoveries were already
well known in the annals of mathematics though unknown to him because he had
not learnt them anywhere; they had been just rediscovered by him. A few of them were even wrong as they showed
him. But most of them were absolutely
new and original and it was now left to these and other mathematical scholars
to make them known in the world of mathematics.
There were many so utterly confounding to them that years and even
decades were to pass before they could be deciphered, understood, and established. In any case, there was a lot more to come
during the following years of collaborative work with Hardy in Cambridge.
Hardy painstakingly edited many of Ramanujan’s manuscripts
giving them the shape, rigour, and professional touch that he was so particular
about, and got them published. He added
his name as a joint author only where he had also made some original
contributions.
Here is a recent picture of the magnificent Trinity College
in Cambridge where the great Newton himself had once worked and on whose
hallowed grounds Ramanujan could now walk proudly as an insider. The same man had no chance whatever of getting
into the Presidency College in Madras a few years earlier.
Ramanujan and Hardy, a
study in contrast
Rarely in the history of intellectual endeavour have two
people of such contrasting backgrounds, characters, styles, and personalities
as Hardy and Ramanujan teamed up to advance the cause of knowledge to the
extent that they did over such a short period of collaboration. Their life and work is a study in contrast
that comes out conspicuously well in Robert Kanigel’s wonderful biography of
Ramanujan and I urge interested readers to go through it.
Stunningly handsome yet a life-long bachelor, aristocratic in
demeanor and cricket crazy, Godfrey Harold Hardy had a soft corner for the
underprivileged (Lenin was one of the men he admired) as the Ramanujan episode
so starkly brings out. As an outstanding
mathematician he was also an uncompromising purist in mathematics, with an
obsessive emphasis on ‘proof’ as the defining characteristic of ‘real’
mathematics. He looked upon applied
mathematics and mathematical physics with some condescending tolerance. He was a devoted atheist (if one can employ
such a description), but never intolerant of conflicting viewpoints. He wielded a considerable influence in the
world of mathematicians, much of it due to the preeminent quality of his work. Above all he could readily recognize and
value any superior intellect. Despite
his own notable discoveries in mathematics, history remembers Hardy principally
for his discovery of Ramanujan.
Srinivasa Ramanujan was the antithesis of Hardy in most respects. He was devoutly religious, believed in and practiced
all the precepts and rituals of his religion and caste, was highly
superstitious, shed his traditional attire only because of the demands of
radically altered circumstances and believed strongly that all his exceptional
abilities were God-given. Being entirely self-taught, he had little idea of how
mathematics was communicated among academicians, had little appreciation for
the concepts of ‘rigour’ and ‘proof’ in mathematics till he interacted with
Hardy, and had little interpersonal contact with other people in
Cambridge. He was well aware that, but
for the benevolence of Hardy in particular, he might not have gained any
professional recognition at all.
Soon after Ramanujan and Hardy met in Cambridge they started
a very fruitful collaboration, each having had a great deal to learn from the
other. They also developed an enormous
respect and admiration for each other even while realizing that they had little
in common at the personal level. In the
matter of beliefs and practices, they carefully avoided treading on each
other’s feet.
One of the best known incidents involving the two is the
episode of the taxicab. While visiting
Ramanujan in his sanatorium years later, Hardy said he had travelled in dull
weather in a taxicab bearing an equally dull number. When asked, Hardy remembered the number to be
1729. Ramanujan flew into a paroxysm of
excitement saying it was anything but dull.
It was in fact the lowest integer known that could be expressed as the
sum of two cubes in two different combinations!
Such was his hold over numbers every one of which was his personal
friend! (After I first read this story
during my school days in Bangalore I realized that the house I was living in
had the same door number as this; however, the house itself was far less
exciting than the number!)
Recognition and Rewards
In March 1916 Ramanujan, who had been a college dropout at
Madras earlier, received the highly valued B A degree of the Cambridge
University based not on any coursework or examination from which he had been
exempted, but on the basis of his published research work on ‘highly composite
numbers’ that was one of his outstanding achievements in Cambridge. Such a degree from Cambridge was easily the
equivalent of a Ph D anywhere else. A
memorable group photograph taken on this occasion (see below) at Trinity
College shows Ramanujan at the centre of a row of students standing stiffly and
uncomfortably, with his mentor G H Hardy providing company at extreme
right. This is one of perhaps just a
handful of photographs showing him that has survived.
Ramanujan was confidently expected to be conferred the even
more highly valued Fellowship of the Trinity College the following year, but
this did not materialize to his great disappointment and of his well-wishers
because of some squabbles within the institution, with an undertone of racism
thrown in. Very sadly, this was also the
beginning of a dark phase in his personal life that was to last the rest of his
time in Cambridge. A mysterious illness,
later diagnosed as tuberculosis, had begun to engulf him. This was to keep him confined to several
sanatoria in England before forcing his departure back home and eventually to
take his life as well. The consequences
of the ongoing First World War in Europe that had left some imprint on
Cambridge too were to exacerbate his situation.
Ignoring the Trinity College fellowship fiasco, Hardy and
eleven other reputed mathematicians decided that Ramanujan was worthy of an
even greater honour – the fellowship of the Royal Society of England – and
nominated him for this. Earlier he had
been elected a fellow of the London Mathematical Society in whose proceedings
quite a number of his papers had been published. Soon enough, Ramanujan received the
enthralling news from Hardy that the Royal Society had indeed decided to confer
him its fellowship in 1918.
Historically, the Fellowship of the Royal Society (FRS) is the highest
academic honour a scientist can get in England and often regarded as next in
importance only to the Nobel Prize. So the college dropout from South India now
became an FRS, one of the youngest ever to get this honour and only the second
Indian to do so. Now that this had
happened, it was easy for Trinity College to follow suit and confer him its own
fellowship that he had been denied earlier – the lesser honour came Ramanujan’s
way after the greater honour. People back home in India were thrilled by
the news and looked upon Ramanujan as their own and an international celebrity,
waiting to bestow their own honours when Ramanujan returned to India.
Last days in England
Even before his health worsened, Ramanujan got into a state
of depression caused by a variety of factors, including his increasing personal
problems both in England and back home in Madras. In one fatefully weak moment he tried to
commit suicide by throwing himself on to a railway track in the London
underground system. An alert railway guard spotted him and brought the train to
a halt before any harm could be done.
The whole episode was hushed up through Hardy’s diplomatic intervention
with the police authorities. Ramanujan
recovered from this incident for some time, buoyed up by the academic honours
that came his way, but the reprieve was short lived. His health deteriorated sharply and he had to
spend time in several sanatoria for tuberculosis patients where the conditions,
particularly the food, were intolerable for him. Hardy advised that it was time for Ramanujan
to return home, at least temporarily, a suggestion acceptable to all concerned
since the world war had also come to an end.
Dying days in India
When he returned to India on 27 March 1919, Ramanujan’s
health had deteriorated to such an extent that his old friends and admirers back
home saw the writing on the wall clearly and tried to prop him up as much as
possible, aided by numerous felicitations and honours showered upon him from
all quarters in Madras, including a professorship at the university which he
had not been able to dream of joining even as a student.
Ramanujan spent his last days in Madras in great agony, both
physical and mental, but his mathematical productivity wasn’t affected
much. Unknown to most people he had been
working on what was to be his magnum opus,
a new and exciting class of functions called ‘Mock Theta’ functions. When he met his end on 26 April 1920 in
Madras, aged just 32, the papers containing these and other discoveries were
found by his wife and passed on to Cambridge through various channels and got
‘lost’ somewhere there, fortunately to be rediscovered decades later.
Commemorating the
genius
Ramanujan’s birth centenary in 1987 and the earlier decades
leading up to this were heralded by numerous events and actions resurrecting and
preserving the memory of this historic personality. Here is a stamp issued by the Indian postal
services to mark his 75th birthday:
Ramanujan’s Kumbakonam residence got a sort of face lift in
2003 and was dedicated to the nation as a memorial by the then president of
India, A P J Abdul Kalam. Its interior houses
a bronze bust of Ramanujan and some memorabilia as seen in the following
picture. It is not clear if the
preservation of the building, changed very little from its original condition,
was an intentional act or one of indifference.
Several international prizes are being offered in memory of
Ramanujan for distinguished original work in mathematics. Among them is the annual ICTP (International
Centre for Theoretical Physics founded in Trieste, Italy by physics Nobel
Laureate Abdus Salaam) Ramanujan Prize for Young Mathematicians from Developing
Countries.
A three-day international conference, ‘ Ramanujan 125,’ was held last month at the Florida State
University in USA to mark the 125th birth anniversary celebration of Ramanujan. Organized by Professors Krishnaswami Alladi
and Frank Garvan of the University of Florida and Ae Ja Yee of Pennsylvania
State University, it brought together about 70 researchers. They delivered talks on current research work
in several areas of mathematics influenced by Ramanujan’s work. The three pre-eminent experts on the
mathematical genius — Professors George Andrews, Bruce Berndt and Richard Askey
all participated.
Ramanujan’s Biography
In 1991, Robert Kanigel, who was not a professional
mathematician but a very competent and experienced science communicator and
professor of science writing at the famed MIT, USA, wrote a voluminous, widely
acclaimed and perhaps definitive biography of Ramanujan after extensive
research on his life and work in both India and England, and titled it “The Man
Who Knew Infinity – A Life of the Genius Ramanujan”. He could more justifiably have changed the
title to “The Man Who Played with
Infinity.” He has perhaps done more to
bring out the authentic genius in Ramanujan than anyone else at the popular
level.
The Ramanujan Scholars
Apart from Hardy, Littlewood and other contemporaries of
Ramanujan, there have been several great names associated with the study of his
monumental notebooks, filling the necessary gaps and publishing the voluminous
results. Three of them, all Americans,
deserve particular mention. They are:
George Andrews of the Pennsylvania University, Bruce Berndt of the University
of Illinois, and Richard Askey of the University of Wisconsin. George Andrews was instrumental in
rediscovering Ramanujan’s long-forgotten and now famous ‘Lost Notebook’ in
Cambridge and making a special study of it, particularly his ‘mock theta’
functions. He and Bruce Berndt together
have brought out a fully edited and elaborated version of the ‘Lost Notebook’. The other four notebooks have all been edited
and brought out by Bruce Berndt. As
Kanigel puts it, these published notebooks today ‘sustain a veritable cottage
industry of mathematicians’ all over the world devoted to their in-depth study.
Postscript
Some of the more prominent discoveries with which Ramanujan’s
name is permanently engraved in the history of mathematics are:
Landau-Ramanujan Constant, Mock Theta Functions, Ramanujan Conjecture,
Ramanujan Prime, Ramanujan-Soldner Constant, Ramanujan theta Function, Ramanujan’s
Sum, Rogers-Ramanujan Identities, and Ramanujan’s Master Theorem. Here is a very small random sample of the
expressions/equations/identities (stated without proof in the original
Carr-inspired style of Ramanujan!) that a student or connoisseur of mathematics
will find exceptionally beautiful and edifying.
To me they are just like listening to the last movement of Beethoven’s
Ninth Symphony, the Ode to Joy.














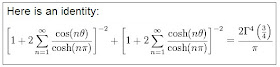




I read the article of The Great Mathematician Rmanujan.I am very much impressed .Even though I donot about the subject. Ur narration is great sir. Thank u.suseela
ReplyDeleteThe article is well written informative and inspiring.
ReplyDeleteHow many people have heard of this genius?? Well I have but because I was not good in geomatry, so, did not follow him much. I read about one particular case, in which, the visitor came in a taxi and he gave some astonishing calculations. Imagine, when Britishers were ruling India, an extremely poor but brilliant person got attention. He moved very late to England because of religious beliefs. Must have been a wonder, considering facilities available at that time and mathmaticians are still working on his theorems.
ReplyDeletegreat to learn about a great man.. had he lived more years world would have seen many changes..
ReplyDelete